POJ 3254 Corn Fields——状态压缩dp

POJ 3254 Corn Fields——状态压缩dp

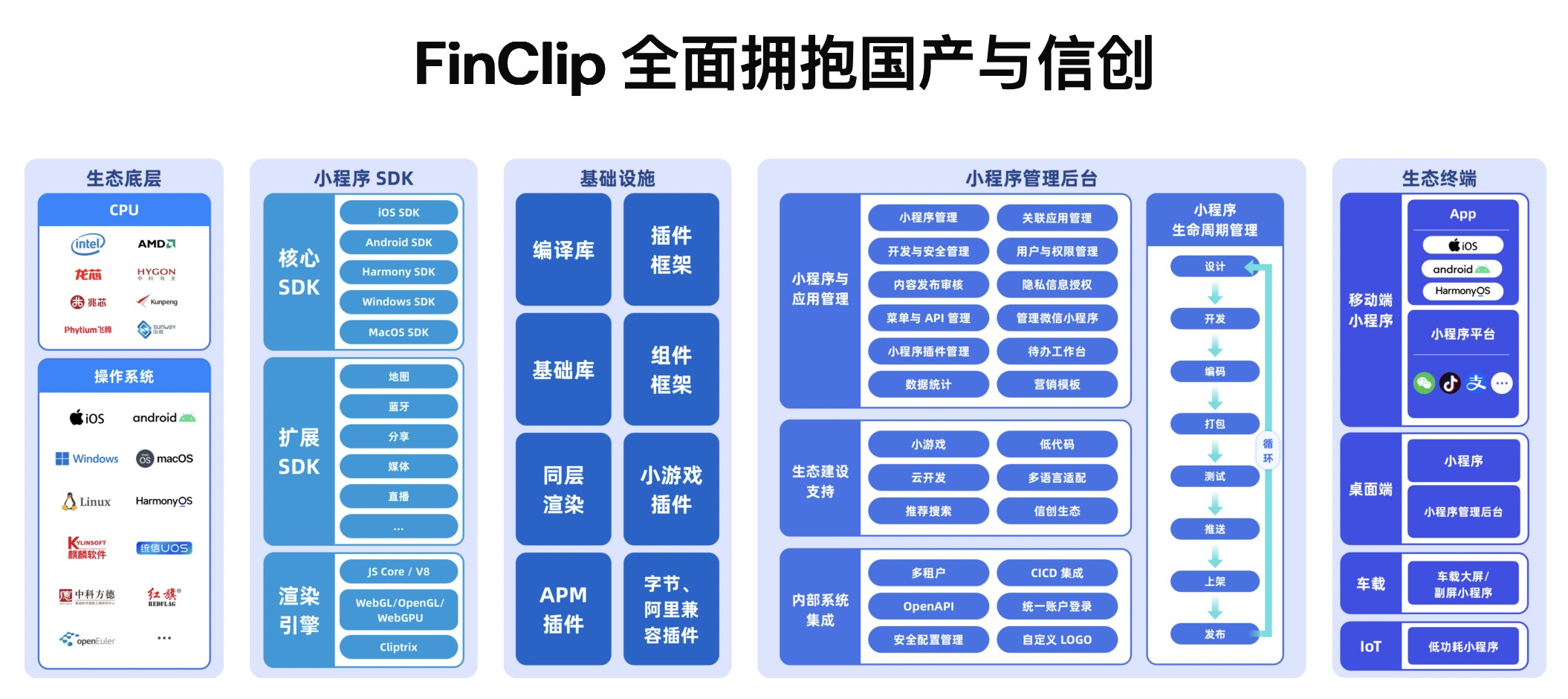
Farmer John has purchased a lush new rectangular pasture composed of M by N (1 ≤ M ≤ 12; 1 ≤ N ≤ 12) square parcels. He wants to grow some yummy corn for the cows on a number of squares. Regrettably, some of the squares are infertile and can't be planted. Canny FJ knows that the cows dislike eating close to each other, so when choosing which squares to plant, he avoids choosing squares that are adjacent; no two chosen squares share an edge. He has not yet made the final choice as to which squares to plant.

Being a very open-minded man, Farmer John wants to consider all possible options for how to choose the squares for planting. He is so open-minded that he considers choosing no squares as a valid option! Please help Farmer John determine the number of ways he can choose the squares to plant.

Input

Line 1: Two space-separated integers:  M and  N  Lines 2..  M+1: Line  i+1 describes row  i of the pasture with  N space-separated integers indicating whether a square is fertile (1 for fertile, 0 for infertile)

Output

Line 1: One integer: the number of ways that FJ can choose the squares modulo 100,000,000.

Sample Input

2 3 1 1 1 0 1 0

Sample Output

9

Hint

Number the squares as follows:

1 2 3   4

There are four ways to plant only on one squares (1, 2, 3, or 4), three ways to plant on two squares (13, 14, or 34), 1 way to plant on three squares (134), and one way to plant on no squares. 4+3+1+1=9.

以row【】记录每行有哪些空位

以state【】记录所有可行状态（即1不连续出现的状态）

以dp【i】【j】记录第i行状态j时的方案数

最后结果就是dp【m】【】的总和

#include #include using namespace std;const int mod = 100000000;int m, n, cnt, row[50], state[1000], dp[50][1000], temp;bool judge1(int x) { if (x & x << 1) return false; return true;}bool judge2(int x, int y) { if (x & row[y]) return false; return true;}void init() { cnt = 0; int total = 1 << n; for (int i = 0; i < total; i++) { if (judge1(i)) state[++cnt] = i; } memset(row, 0, sizeof(row)); memset(dp, 0, sizeof(dp));}int main(){ while (scanf("%d %d", &m, &n) == 2) { init(); for (int i = 1; i <= m; i++) { for (int j = 1; j <= n; j++) { scanf("%d", &temp); if (temp == 0) row[i] += (1<<(n - j)); } } for (int i = 1; i <= cnt; i++) { if (judge2(state[i], 1)) dp[1][i] = 1; } for (int i = 2; i <= m; i++) { for (int j = 1; j <= cnt; j++) { if (!judge2(state[j], i)) continue; for (int k = 1; k <= cnt; k++) { if (!judge2(state[k], i - 1)) continue; if (state[j] & state[k]) continue; dp[i][j] = (dp[i][j] + dp[i - 1][k]) % mod; } } } int ans = 0; for (int i = 1; i <= cnt; i++) { ans = (ans + dp[m][i]) % mod; } printf("%d\n", ans); }}

版权声明：本文内容由网络用户投稿，版权归原作者所有，本站不拥有其著作权，亦不承担相应法律责任。如果您发现本站中有涉嫌抄袭或描述失实的内容，请联系我们jiasou666@gmail.com 处理，核实后本网站将在24小时内删除侵权内容。